About POLA and IPOLA(Summary: Differences between the "ideal" polarisation values for a Graphite Monochromator and "refined" values are expected due to other effects such as Mosaic spread. )
From: "Bob Von Dreele" Subject: Difference between using POLA only and IPOLA/POLA To: Lachlan Cranswick [[email protected]] Date: Wed, 09 Oct 2002 08:58:49 -0700 The two functions are "identical" in effect but differ by a scaling factor. IPOLA=1 is in there to match the "traditional" Azaroff function for diffracted beam monochromators. The difference between theory & measurement for POLA is "expected". The IPOLA=0 function is my adaption so that POLA is the polarized fraction. POLA=0.50 for no polarization and POLA=1.0 for perfect polarization nearly realized in synchrotron radiation & vertical diffraction.
The following two methods show how to setup for the Graphite Monochromator by refining on a suitable standard. In this case, annealed Y2O3. Users of the DBW derived CSIRO Riet7 software or Koalariet would probably feel more at ease using the second IPOLA/POLA method. (In Koalariet, the 2-theta value is inserted).
Click here to obtain GSAS exp and data files of Y2O3 data for determining Polarisation by both methods. (Data from the IUCr CPD Quantitative Phase Analysis Round Robin)
Philips 3020 Goniometer with PW3710 Controller (17.3cm Goniometer Radius).
Copper Long Fine Focus X-ray Tube set at 40kV and 40mA
Flat-plate Bragg-Brentano, reflection geometry
Sample backpacked into standard Philips sample holders
For non Pharmaceutical Samples: Step Scan from 5 to 150,
at 0.02 degree steps and 3 seconds per step
1 degree divergence slit
Incident beam Sollers Slit
Unspun sample
0.3mm receiving slit
Diffracted beam Sollers slit
1 degree scatter slit
Diffracted beam curved Graphite Monochromator
Proportional Counter
002 reflection of Graphite: 2-theta with Cu X-rays = 26.603 (d=3.3480�). Calculated cos^2 2-theta = 0.80
|
Using "POLA only method" to handle a Graphite Monochomator within GSASThe overall advice is to determine the best polarisation value by systematic refinement on an appropriate standard; where data collected up to the angular limit of the Powder XRD (140 to 160 degrees 2-theta). It can be advisable to fit the profile with a Le Bail fit, then fix profile parameters, "shft" offset correction and unit cell values. Be wary that there can be considerable correlation between the polarisation values, thermal values and the background. Using a standard (like cubic annealed Y2O3) where peaks are well separated is advised. First you may want to fix the thermals at reasonable values, then systematically vary the POLA values and see what this does to the R-factors. Then you may want to repeat the above but allow the thermals to vary. Finally, set POLA at extreme values and see if it refines with the thermals to consistent values. If the refinement looks robust, then use this value of POLA for samples generated by this diffractometer. In the following table, POLA consistently refines to a value of 0.523(3) for this particular XRD configuration with a Graphite Monochromator.
Results from refining cubic Y2O3 using the above described strategy
|
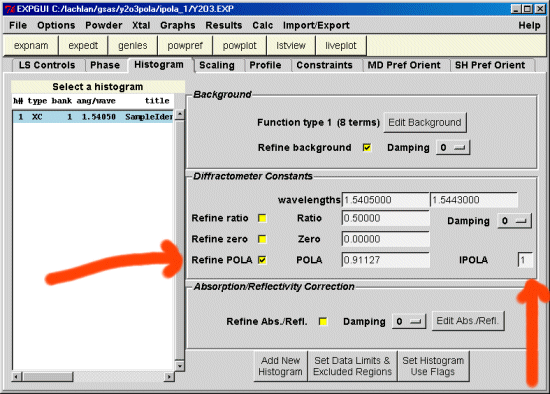
Using POLA and IPOLA=1 to handle a Graphite Monochomator within GSASAs explained above by Bob, set IPOLA to 1 (numeral one), then enter the calculated POLA value as "cos^2 2-theta" for the monochromator. (coefficient in formula for polarisation correction when using a monochromator.) Then for a graphite monochromator, calculate the "cos^2 2-theta" of the 002 graphite reflection (For Cu X-rays this is at an angle of 26.6 degrees 2-theta). The calculated value should be 0.80. Enter this value into the POLA field and refine the structure. Near the end of the refinement, allow POLA to refine (which in this case refines to 0.91(1)). Similarly to the above "POLA only method", it would be best to do similar systematics to ensure you have a robust and reliable result.
|
[Generating and Manipulating Fourier Maps from Powder Data in GSAS]
[Getting back the Forplot Menu after plotting a Windows Fourier Map]
[Generating a VRML based 3D Structure and Electron Density Contour Map in GSAS]
[Viewing GSAS Fourier Contour Maps in 3rd party programs Using Scott Belmonte's GPL'd FOUE software]